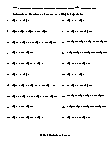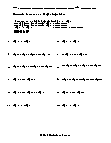Express as a Single Logarithm Worksheets

How to Find Sums and Differences of Logarithms? In the study of algebra, there are many properties you come across with different problems, such as commutative, associative, or distributive properties. The same is the case for logarithm, and there are different properties that let you solve different complex equations easily. Logarithm lets you transform products into sums and quotients into differences. Let us take two examples, each for the sum and difference of logarithms. Now, in this scenario, the above scenario will be separated by using the log property of multiplication. Now, the property of exponents will be applied in the second term, where It will be written first. The next example. This is where we can apply the quotient property and the exponent property of the logarithm.