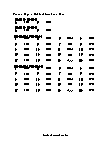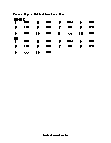Reflection in a Line Worksheets
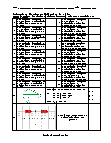
What is a Reflection in a Line? In real life, we consider reflections of images we see when we look in the mirror. Reflection in math, however, follows the same idea. Geometrical reflection correlates with the mirror image in our daily life. Geometrical transformation is used to change the shape and size of a figure or shape. Rigid transformations are those transformations that maintain the length of the shape. Reflection is one of the rigid transformations, that is used to reflect the shape across a defined line. Knowing how to perform reflection of a line is essential for excelling the advanced geometrical skills. In a coordinate plane, we can reflect on the x-axis and the y-axis. A reflection of shape in the x-axis means reflecting an image across the x-axis to obtain a mirror image. In this case, the x-axis is known as the axis of reflection. Similarly, reflection on the y-axis means to reflect a figure or shape across the y-axis to create a mirror image. Here, the y-axis will be known as the axis of reflection. For reflecting the shape across the x-axis, we have to negate every value corresponding to y-coordinate while taking the x-values as same. For instance, a point P lies on (5,4). When reflecting this point across the x-axis we will get the new coordinates as (5, -4). Similarly, when reflecting any figure across the y-axis, we will negate al the x-coordinates and keep y-coordinate the same. For instance, if we reflect the same point P (5,4) across y-axis, we will get, (-5,4).
-
Intermediate Lesson
Demonstrates how to use advanced skills to tackle Reflection in a Line problems.
View worksheet -
Independent Practice 1
A really great activity for allowing students to understand the concepts of the Reflection in a Line.
View worksheet -
Independent Practice 2
Students use Reflection in a Line in 20 assorted problems. The answers can be found below.
View worksheet -
Homework Worksheet
Students are provided with 12 problems to achieve the concepts of Reflection in a Line.
View worksheet -
Basic Lesson
Guides students through identifying reflection in lines based on coordinates.
View worksheet -
Independent Practice 1
A really great activity for allowing students to understand the concepts of the Reflection in a Line.
View worksheet -
Independent Practice 2
Students determine the Reflection in a Line in 20 assorted problems. The answers can be found below.
View worksheet -
Homework Worksheet
Students are provided with 12 problems to achieve the concepts of Reflection in a Line.
View worksheet -
Independent Practice 1
A really great activity for allowing students to understand the concepts of the Reflection in a Line.
View worksheet -
Independent Practice 2
Students use Reflection in a Line in 20 assorted problems. The answers can be found below.
View worksheet -
Homework Worksheet
Students are provided with 12 problems to achieve the concepts of Reflection in a Line.
View worksheet
The Golden Ratio...
The Golden Ratio has fascinated layman and mathematician alike for hundreds of years. It seems like magic, turning up in such different arenas as pine cones, earth-moon and planet relationships, the Cheops Pyramid in Egypt, the Mona Lisa and even our DNA. This widespread appearance exhibits a unifying mathematical principle that is so subtle even science has failed to define it!